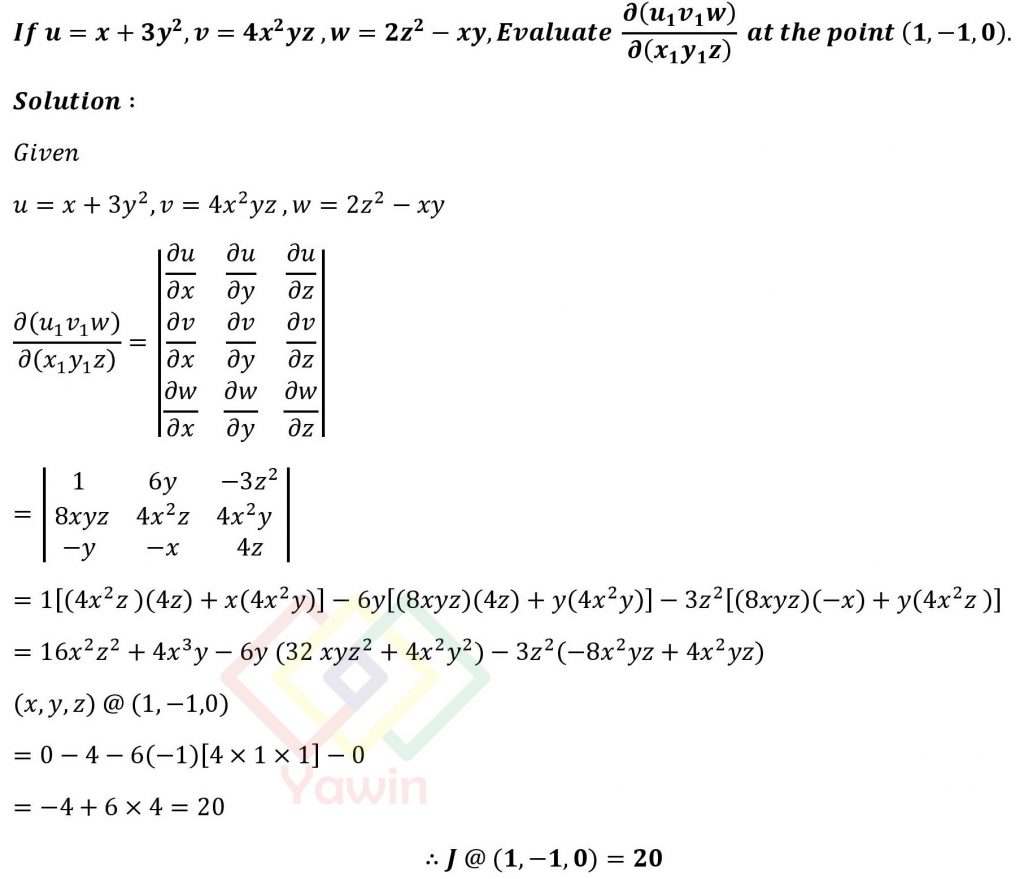
If u=x+3y^2, v=4x^2 yz, w=2z^2-xy, Evaluate del (u,v,w)/del (x,y,z) at the point (1,-1, 0)
Question
Question
If u=x+3y^2, v=4x^2 yz, w=2z^2-xy, Evaluate del (u,v,w)/del (x,y,z) at the point (1,-1, 0)
Jacobian matrix problems
- If u=yz/x, r=zx/y, w=xy/z, Show that J [(u, v, w)/ (x, y, z)] =4
- If u=x+3y^2, v=4x^2 yz, w=2z^2-xy, Evaluate del (u,v,w)/del (x,y,z) at the point (1,-1, 0)
- If u=(x_2 x_3)/x_1, v=(x_1 x_3)/x_2, w=(x_1 x_2)/x_3 find the value of Jacobian J del (u,v,w)/del(x_1,x_2,x_3)
- If u=x^2+y^2+z^2, v=xy+yz+zx, w=x+y+z find the value of Jacobian J del(u,v,w)/ del(x,y,z)
- If u=x+y+z, uv=y+z,uvw=z, then find ∂(x,y,z)/∂(u,v,w)
